Adam Adamandy Kochański
October 4, 2021
I was an early adopter of
desktop computing, and I had an
S-100 bus computer running
CP/M on my desktop long before the introduction of the
first IBM Personal Computer. Before that, I made good use of an
HP-33C Scientific Programmable Calculator for converting
xray diffraction data to
unit cell dimensions of my
epitaxial crystals. This calculator cost about a hundred
dollars when I bought it in 1980. This is $325 in
today's money, but an equivalent calculator can now be purchased for less than ten dollars. The
integrated circuits and
liquid crystal displays of modern calculators operate with much lower
power, and they might derive their power from
photovoltaic cells which were too
inefficient and too expensive in 1980.

I've retained my HP-33C Scientific Programmable Calculator, no longer working, as a keepsake.
This calculator had an display with an eight digit mantissa and two digit exponent formed from red light-emitting diodes.
Nobel Physics Laureate, Kenneth G.Wilson (1936-2013), about whom I wrote in an earlier article (Kenneth G. Wilson, June 21, 2013), remarked about his purchase of an HP pocket calculator.
"I buy this thing and I can't take my eyes off it, and I have to figure out something that I can actually do that would somehow enable me to have fun with this calculator."[1]
(Photo by author.)
This calculator used
reverse Polish notation as a method for data entry. Instead of
keying 1 + 1 to get
2, you would key
1 1 +. While such
stack-oriented calculation made things much easier to implement in
circuitry, I'm certain that the
engineers had a hard time convincing the
marketing people that
customers would adapt. In my case, adaptation was easy, since I had been
programming in
assembly language and
Forth. In the early days of computing, Forth was a good language for
laboratory automation.
Polish notation is named after
Polish mathematician,
Jan Łukasiewicz (1878-1956).
Poland has produced many important scientists and mathematicians. The prime example among the
scientists is
Nicolaus Copernicus (1473-1543), who developed the
heliocentric model of the
Solar System. Also among the early Polish scientists was
astronomer,
Johannes Hevelius (1611-1687), the "founder of
lunar topography," known for his detailed
maps of the lunar
surface that he
published in his
Selenographia, sive Lunae descriptio (Selenography, or a Description of the Moon). Hevelius also discovered four
comets and proposed that comets move in a
parabolic path while near the
Sun.
The
list of Polish mathematicians is voluminous, but I'll mention a few that I recognize through their work.
Adam Adamandy Kochański (1631-1700). Kochański discovered a close approximation of the mathematical constant, pi, that's now called Kochański's approximation. I'll present this later in this article.
Mark Kac (1914-1984). Kac collaborated with Paul Erdős (1913-1996) on the Erdős-Kac theorem. This is an extension of the Hardy-Ramanujan theorem, which is known also as the fundamental theorem of probabilistic number theory. I'll just mention that it involves a term, log(log(n)), and that brings to mind a number theory joke that a drowning number theorist's last words are not, "glug, glug," but "log, log."
Marian Rejewski (1905-1980). Rejewski and colleagues deciphered the German military Enigma cipher machine based on limited documentation from French military intelligence. Over the course of seven years, they continued their decryption, just one step behind modifications to the equipment and the encryption procedures.
Jerzy Różycki (1909-1942). Różycki was a cryptologist who worked with Rejewski on the Enigma cipher.
Wacław Sierpiński (1882-1969).
Sierpiński is known for the Sierpiński triangle (a.k.a., the Sierpiński gasket).
I wrote about this is an earlier article (Patterns from Randomness, May 18, 2017).
(Wikimedia Commons image. Click for larger image.)
Alfred Tarski (1901-1983). Tarski and Stefan Banach (1892-1945) created the Banach-Tarski paradox that states that a solid sphere in three-dimensional space can be decomposed and reconstructed into two identical copies of the original sphere. This is as strange as anything in quantum mechanics.

It's all clear now - Humpty Dumpty was just trying to clone himself! This is an artistic representation of the Banach-Tarski paradox in which a solid sphere can be broken apart and reconstructed into two identical copies of itself.
(Wikimedia Commons image by Benjamin D. Esham.)
Stanisław Ulam (1909-1984). In the late 1940s, Ulam invented the Monte Carlo method that I've used often. His inspiration came from his playing the solitaire card game while recovering from surgery. He realized that computers could be used to play many "games" to statistically estimate the outcome of physical processes.
Józef Hoëné-Wroński (1776-1853). The Wrońskian is a determinant that's used to show linear independence in a set of solutions.
Polish mathematician, Adam Adamandy Kochański, was also a
physicist, a
clockmaker, a
Jesuit, and the
Chaplain and
Court Mathematician of
John III Sobieski, the
King of Poland. Alas, the
United States has a
Surgeon General, but no Court Mathematician. His best known work is his 1685
Observationes Cyclometricae ad facilitandam Praxin accommodatae (Cyclometric Observations to Facilitate the Process of Estimation), which considers the
ancient problem of
squaring the circle. This was published in
Acta Eruditorum, the leading
scientific journal of his time. Squaring the circle will give a value for pi, and his method leads to a very close value called Kochański's approximation; viz,[2]
√((40/3)-2(√3)) = 3.14153333870509461863...
Which is
accurate to four
decimal places (pi is 3.1415926535...). While pi is known today to 50 trillion decimal places (5 x 10
13), this was a rare achievement in his time.
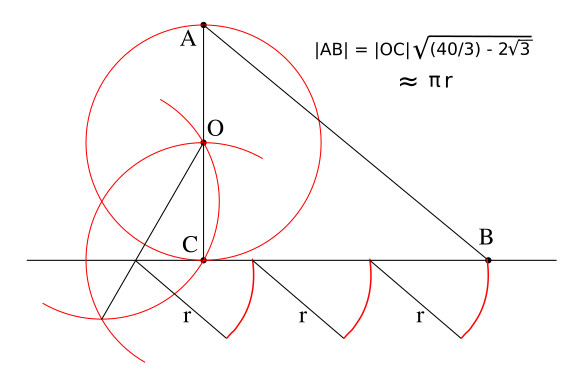
Kochański's geometric construction to get a close approximation of the mathematical constant, pi. In the above figure, let the circle radius be one. The circular arc drawn with radius 1 at C intersects the circle at point (-√(3)/2,1/2). At that point, we draw another circular arc of radius 1, and the two arcs intersect at (-√(3)/2,-1/2). We construct the line through that intersection and the circle origin, and this intersects the perpendicular line drawn at C to produce the point at (-√(3)/3,0). Going three radii along this line gives point B at (3-√(3)/3,0), and the length of AB is √(22+(3-(1/3)√(3))2)=√((40/3)-2√(3)).[2] (Highly modified version of a Wikimedia Commons image. Click for larger image.)
References:
- Martin Weil, "Kenneth Wilson, Nobel winner who explained nature's sudden shifts, dies in Maine at 77," Bangor Daily News, June 19, 2013.
- Eric W. Weisstein, "Kochanski's Approximation." From MathWorld--A Wolfram Web Resource.
Linked Keywords: Desktop computer; desktop computing; S-100 bus; CP/M; IBM Personal Computer; HP-33C Scientific Programmable Calculator; xray crystallography; xray diffraction; data; lattice constant; unit cell dimension; epitaxy; epitaxial crystal; dollar; inflation; today's money; integrated circuit; liquid crystal display; electric power; solar cell; photovoltaic cell; energy conversion efficiency; inefficient; souvenir; keepsake; seven-segment display; significand; mantissa; floating point; exponent; light-emitting diode; Nobel Physics Laureate; Kenneth G.Wilson (1936-2013); reverse Polish notation; computer keyboard; key; stack (abstract data type); electronic circuit; circuitry; engineer; marketing; customer; computer programming; assembly language; Forth (programming language); laboratory automation; Polish notation; Poland; Polish; mathematician; Jan Łukasiewicz (1878-1956); timeline of Polish science and technology; scientist; Nicolaus Copernicus (1473-1543); heliocentrism; heliocentric model; Solar System; astronomer; Johannes Hevelius (1611-1687); Moon; lunar; topography; map; regolith; surface; publish; Selenographia, sive Lunae descriptio (Selenography, or a Description of the Moon); comet; parabola; parabolic; orbit; path; Sun; list of Polish mathematicians; Adam Adamandy Kochański (1631-1700); approximation; mathematical constant; pi; Mark Kac (1914-1984); collaboration; collaborated; Paul Erdős (1913-1996); Erdős-Kac theorem<; Hardy-Ramanujan theorem; fundamental theorem; probabilistic number theory; joke; drowning; number theorist; last words; Marian Rejewski (1905-1980); cryptanalysis; decipher; Germany; German; military; Enigma cipher machine; documentation; France; French; military intelligence; decryption; encryption; Jerzy Różycki (1909-1942); cryptologist; Enigma cipher; Wacław Sierpiński (1882-1969); Sierpiński triangle (a.k.a., the Sierpiński gasket); Wikimedia Commons; Alfred Tarski (1901-1983); Stefan Banach (1892-1945); Banach-Tarski paradox; solid geometry; sphere; three-dimensional space; decomposition; decompose; reconstruct; quantum mechanics; Humpty Dumpty; cloning; clone; artistic representation; Benjamin D. Esham; Stanisław Ulam (1909-1984); 1940s; invention; invented; Monte Carlo method; Eureka effect; inspiration; solitaire; playing card; card game; surgery; statistics; statistical; estimation; estimate; physics; physical processes; Józef Hoëné-Wroński (1776-1853); Wrońskian; determinant; linear independence; equation; solution; physicist; clockmaker; Society of Jesus; Jesuit; Chaplain; royal court; John III Sobieski; King of Poland; United States; Surgeon General of the United States; ancient; mathematics problem; squaring the circle; Acta Eruditorum; scientific journal; accuracy and precision; accurate; decimal place; geometric construction; approximation; mathematical constant; pi; radius<; circular arc; intersection (Euclidean geometry); intersect; point )geometry); geometric construction; line (geometry); perpendicular; length.