Squeeze Bottles
January 30, 2023
Dripping water faucets were a curse upon every
household before the development of better
elastomers to replace
natural rubber for faucet
washers and
gaskets. There are now
washerless faucets designed with closely fitting
ceramic disks with
triangular cutouts that limit the water
flow and don't need washers.

A water drop striking the bottom of a metal kitchen sink.
There are a few techniques to mitigate the annoying sound of the water drops from a dripping water faucet before the washer is replaced.
You can wrap a towel around the faucet and direct the other end into the drain; attach a piece of string, sewing thread, or dental floss, to the faucet so that the drip slides down the fiber into the drain; or, place a tall bottle under the faucet so that the water slides down the outside of the bottle.
For a double basin sink, direct the drips to slide down the basin divider; or, submerse the faucet in a tall tumbler filled with water. The excess water from the drips will slide down the side of the container.
(Wikimedia Commons image by Omegatron)
Water drips can be useful.
Water clocks in which water drips from one container to another to mark the passage of
time were used by people in
antiquity. The
ancient Greeks named these
clepsydra, but water clocks were found in many other
cultures, such as
Egypt,
Babylon, and
Persia, as far back as the
16th century BC. The
Roman engineer and
architect,
Vitruvius (c. 80–70 BC - c. 15 BC), wrote about water clocks in Book IX, Chapter 8, of his
De architectura.[1]
As anyone who has used a large
funnel has noticed, the
flow rate through the
orifice is rapid when the
liquid level is high, and it slows at the end of its
discharge. That's because liquid at the top
falls from a higher
height, and its
gravitational force gives a higher
pressure at the exit orifice, a
principle known as
hydraulic head. This
phenomenon was
quantified by
Italian physicist,
Evangelista Torricelli (1608-1647).
Torricelli's law gives the
speed at which a
fluid exits a
hole in a container as a
function of the height of the fluid above the hole. According to this law, the exit
speed v is given as
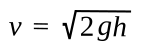
where
g is the
gravitational acceleration, about 9.8 m/sec
2 at
Earth's surface, and
h is the height of the fluid above the hole. A
decade ago I
experimented with the
design of a
digital water clock, the basic principle of which is shown in the figure. An
optical interrupter is connected to a
microcontroller to mark the passage of time by counting the water droplets as they fall through the
gap. The drop rate is the equivalent of the flow rate, and a
data analysis to verify Toricelli's Law is straightforward.
For the
data shown in the figure, the container held about three
cups of water (about 700
mL), the initial fluid level was four
inches, and it was reduced to one inch over the course of the experiment. As can be seen, Torricelli's Law is verified over this small change in fluid height as a
straight line fit when the
drop rate is
plotted against the
square-root of the fluid height.
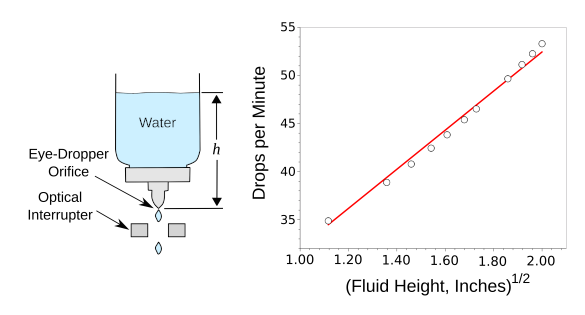
On the left is a schematic diagram of the water drop assembly for the water clocks. The optical interrupter sensed the passage of each water drop. On the left is data collected that verifies Torricelli's law by showing a straight line fit when the drop rate is plotted against the square-root of the fluid height. (Left image created using Inkscape. The data on the right graphed using Gnumeric. Click for larger image.)
My water clock was to have solved the problem of changing drop rate as water is lost by
pumping water from a
basin back to the upper reservoir. I even designed a
circuit to
synthesizer a
bloop sound to mark the passage of each drop. I used
distilled water to prevent anything from
clogging the dropper orifice. Unfortunately, the drop rate unexpectedly diminished over time, a phenomenon that I attributed to
microscopic air bubbles adhering to the inside of the dropper orifice to restrict the water flow.
room temperature water is a low
viscosity Newtonian fluid with
mathematically simple fluid flow behavior. Common kitchen
condiments, such as
ketchup,
mustard, and
mayonnaise, are
non-Newtonian fluids whose viscosity will change when under force to become less viscous and more liquid. Such condiments are sold in convenient
squeeze bottles; and, as most of us know,
squeezing that last
portion from such bottles often leads to an
explosive splatter.

Would you prefer ketchup, or catsup, on your French fries?
The popular tomato condiment was generally called catsup in the past, but ketchup is presently the preferred spelling.
These are three of the squeeze bottles in my refrigerator; so, you now know my brand preferences. I should switch to glass bottles, since glass is more likely to be properly recycled than plastic.
(Photo by the author.)
Callum Cuttle and
Christopher MacMinn of the
Department of Engineering Science at the University of Oxford performed
experiments on this effect.[3-5] They presented their
research at the
American Physical Society's Division of Fluid Dynamics conference (Indianapolis, Indiana) in
November of last year,[4] and a
preprint of their research is avaibale at
arXiv.[3]
When bottles like the ones shown above are squeezed, the
air in their inner
volume is
compressed, this pushes the viscous contents through the exit orifice, but the
material's viscosity resists its flow.[4] The Oxford University
engineers created an idealized experiment in which air is injected by a
syringe pump into a
capillary tube filled with
silicone oil (see figure).[3-4]
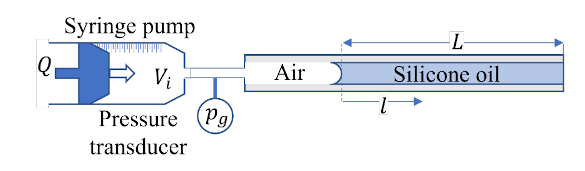
In the experiments, the displacement of silicone oil from a capillary tube is caused by the injection of air from a reservoir of initial volume Vi that's compressed at a constant volume rate Q by a syringe pump. The displacement of the interface as a function of time l(t) relative to its initial position (l(0) = 0) and the gauge pressure pg of the gas are measured. The initial length of the silicone oil region is L. (Fig. 1(a) of ref. 3.)[3]
Although the experimental system is simple, it does
replicate the essential properties of this type of flow.[4] It was found that this process could be
modeled by a single
dimensionless compressibility number, which depends things such as the amount of air, the
diameter of the exit orifice, and the rate of air compression.[3-4] A
critical value of this compressibility number defines a
transition between two
regimes, with the high-compressibility regime showing the burst-like
expulsion with which many of us are familiar.[3]
As paper
co-author, Callum Cuttle, explains,
"Our analysis reveals that the splattering of a ketchup bottle can come down to the finest of margins: squeezing even slightly too hard will produce a splatter rather than a steady stream of liquid."[4]
To avoid the splatter, you could squeeze the bottle more slowly to reduce the rate of gas compression. Howver, the most effective technique would be a bottle designed with a larger
diameter exit orifice, since the larger diamter results in a larger
area that reduces the
viscous drag.[4] Says Cuttle, the main
culprit is the
rubber exit
valve.
"These valves make the spattering problem worse by forcing you to build up a certain amount of pressure before the sauce can even start to escape. These valves help to avoid leaks, but purely from a splattering perspective, removing these valves would make a lot of sense. For a quick remedy, when you get close to the end of a bottle (when a splatter is most likely), just take the cap off and squeeze the remaining liquid out of the broader neck. It's common sense, but now there is a rigorous mathematical framework to back it up."[4]
Beyond the kitchen, this research can have application in other areas that involve displacement of a fluid with a gas.[4] These include the
storage of captured carbon dioxide in
aquifers, some types of
volcanic eruption, the
re-inflation of
collapsed lungs, and in
fuel cells.[4]
References:
- Marcus Vitruvius Pollio, "de Architectura," Book IX, on Bill Thayer's University of Chicago website .
- Ketchup, catsup, catchup, grammarist.com.
- Callum Cuttle and Christopher W. MacMinn, "Dynamics of compressible displacement in a capillary tube," arXiv, December 24, 2021, https://doi.org/10.48550/arXiv.2112.12898.
- Mathematical model could help avoid spattering squeezy sauce bottles, University of Oxford Press Release, November 16, 2022.
- Jennifer Ouellette, "Oxford scientists crack case of why ketchup splatters from near-empty bottle," arstechnica.com, November 24, 2022.
Linked Keywords: Drop (liquid); drip; dripping; tap (valve); water faucet; household; elastomer; natural rubber; washer (hardware); gasket; ceramic; disk (mathematics); triangle; triangular; fluid dynamics; flow; water; drop (liquid}; impulse (physics); strike; metal; kitchen; sink; mitigate; annoyance; annoying; sound; towel; drain (plumbing); fiber; string; thread (yarn); sewing thread; dental floss; bottle; double basin sink; tumbler (glass); container; Wikimedia Commons; Omegatron; water clock; time; ancient history; antiquity; ancient Greece; ancient Greeks; clepsydra; culture; Egypt; Babylon; Iran - classical antiquity; Persia; 16th century BC; Roman Empire; engineer; architect; Vitruvius (c. 80–70 BC - c. 15 BC); De architectura; funnel; volumetric flow rate">flow rate; nozzle; orifice; liquid; discharge (hydrology); altitude; height; gravitation; gravitational force; pressure; physical law; principle; hydraulic head; phenomenon; quantification (science); quantify; quantified; Italy; Italian; Evangelista Torricelli (1608-1647); Torricelli's law; speed; fluid; hole; function (mathematics); gravitational acceleration; lithosphere; Earth's surface; decade; experiment; experimented; design; digital; slotted optical switch; optical interrupter; microcontroller; gap; data analysis; data; cup (unit); SI prefixes applied to the litre; milliliter; inch; line (geometry); straight line; regression analysis; fit; plot (graphics); plotted; square-root; Toricelli Law experiment; schematic diagram; water; assembly; sensor; sense; reproducibility; verify; Inkscape; Gnumeric; pump; pumping; bowl; basin; electronic circuit; analog synthesizer; distilled water; clog; clogging; microscopic scale; bubble (physics); air bubble; adhesion; adhering; room temperature; viscosity; Newtonian fluid; mathematics; mathematically; condiment; ketchup; mustard (condiment); mayonaisse; non-Newtonian fluid; squeeze bottle; compression (physics); squeezing; serving size; portion; explosion; explosive; splatter; catsup; French fries; tomato; spelling; refrigerator; brand; preference; glass; recycling; recycled; plastic; Callum Cuttle; Christopher MacMinn; Department of Engineering Science; research; American Physical Society's Division of Fluid Dynamics conference (Indianapolis, Indiana); November; preprint; arXiv; atmosphere of Earth; air; volume; compression (physical); compressed; material; syringe driver; syringe pump; capillary action; capillary tube; silicone oil; displacement (fluid); interface (matter); function (mathematics); time; position (vector); pressure measurement; gauge pressure; gas; measurement; measured; reproducibility; replicate; mathematical model; modeled; dimensionless quantity; compressibility; number; diameter; critical; transition; regime; expulsion; co-author; analysis; area; drag (physics); low Reynolds number; Stokes' drag; viscous drag; culprit; valve; sauce; leak; bottle cap; common sense; mathematical proof; rigorous mathematical framework; storage of captured carbon dioxide; aquifer; volcanic eruption; re-inflation; pneumothorax; collapsed lung; fuel cell.