Knots
January 24, 2020
Nobel Laureate,
Werner Heisenberg, and I have at least one thing in common - We were both
boy scouts. I was in the
Boy Scouts of America for two years during
elementary school, and I even spent two weeks at a scout
summer camp where we did such things as
swimming,
hiking, and
reforestation. I even started a
campfire from a
spark made by
striking flint rock to
steel. Heisenberg was a member of the
Bund Deutscher Neupfadfinder, the New Boy Scouts, a part of the traditional
German Youth Movement.
One scout activity that I remember is
knot tying, an important part of which is learning the distinction between a
square knot (also called a
reef knot), and a
Granny knot. The Granny knot likely got its name name from being a common
sack-tying knot in
granaries, but the
idea that it was an inferior knot favored by
grandmothers reinforced the
concept in a young scout that it should be avoided. The Boy Scouts was also the place that I discovered that you don't need two pieces of
rope to tie a knot - You use each end of a single piece as two different ropes. That
revelation may have subtly influenced some of my later
scientific thinking.

A square knot and Granny knot. The difference is subtle, but the granny knot slips when loaded, while the square knot does not. A common mnemonic phrase for tying a square knot is, "Right over left, left over right, makes a knot both tidy and tight." There are many other knot types, and this YouTube video demonstrates seven of them. (Created using Inkscape from Wikimedia Commons source files).
The
holiday season is not that far behind us, so most of us have recently experienced the
chaotic knotting found in the
tangle of
holiday light strings. While it's not surprising to learn that
mathematicians have developed the idea of
the knot into a mathematical object, it is surprising to learn that
physicists have investigated tangled strings as physical objects.[1-3] In 2007, physicists from the
University of California at San Diego published the results of their
experiments on the spontaneous formation of knots in a piece of
string.[1-2]. Their simple experiment consisted of dropping a string into a
box,
rotating the box at about one
revolution per
second for ten seconds, and repeating this operation for 3,415 trials in which they varied the
length of the string, the
stiffness of the string, the size of the box, and the rotation rate.[1-2]
The experiments showed that the highest
probability of knotting was for a long,
flexible string in a large box, since a small box limits the possible string motions. It's
intuitive that a stiff string won't bend as much, which will reduce the knotting probability; and, of course, a long string gives a greater probability of overlaps that lead to knotting. Strings less than about 18
inches did not form knots, and the knotting probability increased sharply after 18 inches, leveling-off at five
feet.[1] One of the experimenters, Raymer, developed a knotting
model in which a string will form
concentric loops when dropped into the box, and rotation allows the free ends to
weave through the loops.[1-2]
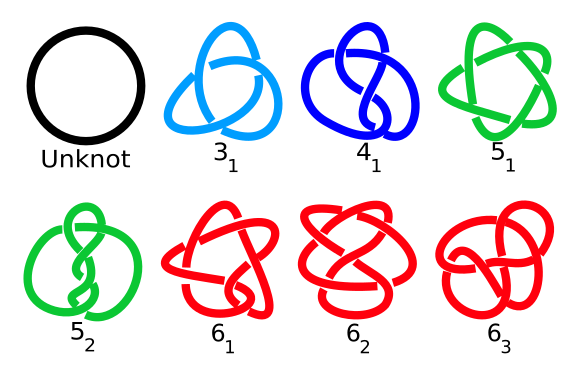
The knot as a mathematical object.
These knots are labeled using the Alexander-Briggs notation, introduced in a 1927 paper by mathematicians, James W. Alexander and Garland B. Briggs.
The important number here is the first, which is the crossing number. The second is an arbitrary number assigned to members of that crossing.
(Based on a Wikimedia Commons image by Jkasd. Click for larger image.)
While knots are all too common and often
frustrating in our lives, there is only one major
myth involving a knot. That's the supposedly
untieable Gordian Knot, for which there was a
prophesy that the person who could untie it would become the
king of
Asia Minor. If this prophesy sounds familiar, it was likely the basis of the
legend of
Arthur pulling the
sword,
Excalibur, from a
stone to become king of
Britain.
Alexander of Macedonia (a.k.a.,
Alexander the Great) untied the knot in 333 BC, by either cutting it with his sword, or sliding the knot off the post to which it was tied, and he proceeded to conquer Asia Minor.
Further
research on knots has recently been undertaken by a team of mathematicians and
mechanical engineers at the
Massachusetts Institute of Technology (MIT, Cambridge, Massachusetts).[4-5] Using
data from experiments in which
color-changing
fibers revealed the localized
stress in knots as they are pulled apart and
computer simulations of these processes, they developed a
theory of knot stability based on the
topological parameters of
twist charge, crossing numbers, and
handedness. The theory predicts why some knots (like the granny knot) will slip easily and untie, whereas others (like the square knot) will tightly hold.[4-5] They report their results in a recent issue of
Science.[4]
Mathematical knots are
abstract objects disconnected from the
physical properties of actual knots, and there has been no theoretical work on what makes the better knot. As
Mathias Kolle, an
associate professor at MIT and a member of the research team explains, "
Empirical knowledge refined over
centuries has
crystallized out what the best knots are," so the MIT research team decided to develop a mathematical model of knots that would explain why one knot is
stronger than another.[5]
Kolle's MIT research group produced
stretchable fibers that change color in response to
pressure or
strain in 2018.[5] Kolle, who is an avid
sailor, was no stranger to knots, so he and his team
photographed pulled knots made from such fibers to deduce their
mechanical state.[5] As confidence built for their experimental approach, they did experiments and computer simulations for more complicated knots and were able to categorize knots according to their relative strength and key properties that included the number of fiber crossings and the direction in which the fiber segments twist as the knot is tightened.[5] These properties are
analogous to those that describe
long-range ferromagnetic spin systems.[4]

Simple knot in a fiber made from a material that changes color according to stress state.
Data from such fibers in knot tying experiments allowed MIT researchers to develop a mathematical model for prediction of a knot's stability.
(MIT image, licensed under a Creative Commons Attribution Non-Commercial No Derivatives license. Click for larger image.[5])
Says
Jörn Dunkel, research team member and associate professor of mathematics at MIT,
"These subtle differences between knots critically determine whether a knot is strong or not... With this model, you should be able to look at two knots that are almost identical, and be able to say which is the better one."[5]
It was found that a knot is stronger if it has more fiber crossings.[5] It is likewise stronger if it contains a greater number of so-called
twist fluctuations, which are changes in the direction of rotation from one fiber segment to another.[5] Stronger knots have more
circulations which are regions in which two
parallel fibers loop against each other in opposite directions.[5] Such rules explain why a square knot is stronger than a granny knot, since the square knot has a higher number of twist fluctuations.[5] As Kolle summarizes,
"If you take a family of similar knots from which empirical knowledge singles one out as the best, now we can say why it might deserve this distinction... We can play knots against each other for uses in suturing, sailing, climbing, and construction. It's wonderful."[5]
This research was supported in part by the
Alfred P. Sloan Foundation, and the
National Science Foundation.[5]
References:
- Dorian M. Raymer and Douglas E. Smith, "Spontaneous knotting of an agitated string," Proc. Natl. Acad. Sci., vol. 104, no. 42 (October 16, 2007), pp. 16432-16437, https://doi.org/10.1073/pnas.0611320104.
- Jeanna Bryner, "The Science of Knots Unraveled," LiveScience, October 3, 2007.
- Andrew Belmonte, "The tangled web of self-tying knots," Proc. Natl. Acad. Sci., vol. 104, no. 44 (October 30, 2007), pp. 17243-17244, https://doi.org/10.1073/pnas.0708150104.
- Vishal P. Patil, Joseph D. Sandt, Mathias Kolle, and Jörn Dunkel, "Topological mechanics of knots and tangles," Science, vol. 367, no. 6473 (January 3, 2020), pp. 71-75, DOI: 10.1126/science.aaz0135.
- Jennifer Chu, "How strong is your knot?" MIT Press Release, January 2, 2020.
Linked Keywords: Nobel Prize in Physics; Nobel Laureate; Werner Heisenberg; scout; scouting; boy scouts; Boy Scouts of America; elementary school; summer camp; swimming; hiking; reforestation; campfire; spark (fire); impact (mechanics); strike; flint rock; fire striker; steel; Bund Deutscher Neupfadfinder; German Youth Movement; knot; square knot; reef knot; Granny knot; flour sack; granary; idea; grandparent; grandmother; reinforcement; reinforce; concept; rope; revelation; science; scientific; structural load; loaded; mnemonic; phrase; YouTube video; Inkscape; Wikimedia Commons; Christmas and holiday season; chaos; chaotic; tangled; tangle; Christmas lights; holiday light string; mathematician; knot into a mathematical object; physicist; University of California at San Diego; scientific literature; publish; experiment; twine; string; box; rotation; rotate; revolution; second; length; stiffness; probability; flexible; intuition (philosophy); intuitive; inch; foot (unit); feet; mathematical model; concentric; weaving; weave; Alexander-Briggs notation; mathematician; James W. Alexander; number; arbitrariness; arbitrary; Jkasd; frustration; frustrating; myth; untieable; Gordian Knot; prophesy; monarch; king; Asia Minor; Matter of Britain; legend; King Arthur; sword; Excalibur; rock (geology); stone; Britain; Alexander of Macedonia; Alexander the Great; research; mechanical engineering; mechanical engineer; Massachusetts Institute of Technology (MIT, Cambridge, Massachusetts); data; color; fiber; stress (mechanics); computer simulation; theory; topology; topological; parameter; twist (mathematics); twist charge; chirality (mathematics); handedness; Science (journal); abstraction; abstract; physical property; Mathias Kolle; associate professor; empirical evidence; empirical knowledge; century; centuries; crystallize; stretch; pressure; deformation (mechanics); strain; sailor; photograph; mechanics; mechanical; analogy; analogous; order and disorder (physics); long-range; ferromagnetism; ferromagnetic; Spin (physics); material; prediction; Creative Commons Attribution Non-Commercial No Derivatives license; Jörn Dunkel; parallel (geometry); surgical suture; sailing; climbing; construction; Alfred P. Sloan Foundation; National Science Foundation.