Bio-Inspired Electrode
May 7, 2018
Along with the obligatory
calculation of the lengths of the sides of a
triangle, students of
trigonometry learn how to calculate the
areas of simple
polygons. The area of simple
planar objects, such as
circles, triangles, and
rectangles has been known for more than two
millennia, and
Archimedes (c.287 BC - c.212 BC) determined the
surface area of a sphere in 225 BC in his
manuscript,
On the Sphere and Cylinder. It's interesting that finding the surface area of a sphere is a common exercise for students of
calculus, an area of
mathematics that was developed two thousand years after Archimedes.
The usual
formula for calculating the area of a triangle is taking half the product of its
base and
altitude. This, of course, requires first calculating the altitude. That's why I was happy when I discovered
Heron's formula for calculating the area of an arbitrary triangle from the length of its sides. I wrote about Heron's formula in a
previous article (Random Triangles, January 26, 2017).
Hero of Alexandria (c. 10 - 70 AD), more properly, Heron, was both a mathematician and an engineer, having invented quite a few devices, including a steam engine, called the Aeolipile.
(Illustration from the Codex of San Gregorio de Nizance, a ninth century Greek manuscript, via Wikimedia Commons.)
Hero's simple formula for calculating the area
A of an arbitrary triangle from the length of its sides appears in his Metrica (c. 60 AD).[1] Using
a,
b, and
c as the length of the sides, the formula is as follows:
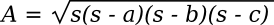
Where the
parameter, s, known as the
semiperimeter, is half the
perimeter; viz.,
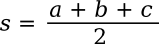
If you want to eliminate the semiperimeter from the formula, the formula can be written in terms of the sides, only, as
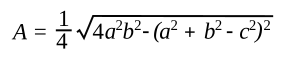
Decades ago, surface area measurement of
materials was generally important for just one thing,
catalysis. Since
heterogeneous catalysis involves the disassociation and reassembly of
gas or
liquid molecules on the surface of a
solid, it's important in
research to know how much surface is available for catalysis. There's the additional research goal of trying to maximize the surface area of a particularly good
catalyst.
One easy way to determine the surface area of a solid is to measure the quantity of an
adsorbed gas and relate that to the area occupied by one gas molecule. This method was pioneered by
Nobel Laureate Irving Langmuir (1881-1957) who developed what's called the
Langmuir isotherm in 1916. Langmuir was awarded the
Nobel Prize in Chemistry in 1932 "for his discoveries and investigations in surface chemistry."
Hitting the books at an early age can lead to a Nobel Prize at a later age, as evidenced by Irving Langmuir (1881-1957).
Langmuir spent much of his working life at the General Electric Research Laboratory, Schenectady, New York, starting there in 1919.
Science fiction author, Kurt Vonnegut (1922-2007), who worked as a publicist at the General Electric Research Laboratory in his youth, said that Langmuir was the inspiration for his fictional inventor of ice-nine (not to be confused with an actual phase of water, ice-IX), that eventually crystallized all the water in the world.
(Left image and right image via Wikimedia Commons, modified for artistic effect.)
Langmuir's simple model of
adsorption, which concerned just gas adsorption on a surface, supposed that gas molecules would stick to the surface by
chemical adsorption or
physical adsorption. He also supposed that the adsorbed film would be one molecule thick. He calculated that at a constant
temperature (thus, the
isotherm designation) the surface coverage
θA would follow the simple expression,
θA = P/(P + P0), in which
P is the
partial pressure of the gas, and
P0 is a critical pressure. The
graph illustrates how the surface coverage levels off at pressures greater than
P0, in this example, 50.
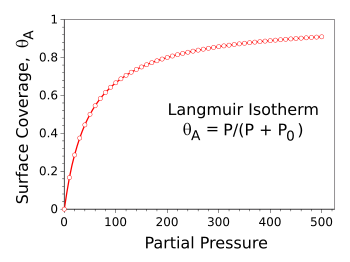
Langmuir isotherm for P0 = 50.
The Brunauer–Emmett–Teller (BET) isotherm is often used in place of the Langmuir isotherm, since it gives better results for physical adsorption of inert gases. It was developed by chemists, Stephen Brunauer (1903-1986) and Paul Hugh Emmett (1900-1985), and physicist, Edward Teller (1908-2003).
It's interesting to note that Brunauer was forced to resign from his position with the US Navy as a consequence of McCarthyism, while his co-author, Teller, was at the opposite end of the political spectrum in his opinion that J. Robert Oppenheimer (1904-1967) should be denied a security clearance.
(Created using Gnumeric. Click for larger image.)
The active surface area of a material has become important in the optimization of
electrodes for
battery and
supercapacitor energy storage. When we examine the relevant equations for a
parallel plate capacitor, we see that the stored energy
W is directly related to the electrode area
A; viz.,
C = κεoA/d
W = (1/2) C V2
where
C is the
capacitance,
κ is the
dielectric constant,
εo is the
permittivity of free space (8.854 x 10
-12 farads per
meter),
d is the plate separation, and
V is the
voltage. With farads as the units of capacitance, the stored energy will have units of
joules.
Electrode area in
electric double-layer supercapacitors is enhanced by the use of very high area electrodes made from
activated charcoal. As shown in the figure, a thin dielectric layer is formed between
conductive electrolyte layers. This results in many farads of capacitance in a few
cubic inches; however,
electrochemical decomposition of the electrolyte limits operation to about 3 - 5
volts.
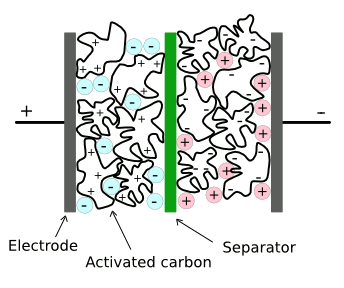
Structure of a conventional supercapacitor.
There are separate ionic liquids that are negatively and positively charged. One disadvantage of current materials is that these ionic liquids decompose at voltages above a few volts. (Via Wikimedia Commons, modified))
Activated charcoal is easy to make, it's
inexpensive, and it can be used as an effective high surface area electrode. However, a little more effort can create
carbon electrodes of even greater surface area. A team of
scientists and
engineers from
Purdue University (West Lafayette, Indiana), the
University of Nevada (Reno, Nevada),
Central South University (Changsha, China),
Mississippi State University (Starkville, Mississippi),
Yangtze University (Jingzhou, China), and the
University of California (Los Angeles, California) have developed a
bio-inspired leaves-on-
branch hybrid carbon
nanostructure for supercapacitor electrodes. They've reported their results in an
open access paper in
Nature Communications.[2-3]
Carbon nanomaterials such as
carbon nanotubes,
nanofibers, and
graphene, have been investigated as a way to enhance the energy density of electrodes since they have high surface area and are
electrically conductive.[2] Highly-ordered carbon nanomaterials have better properties in electrode applications than
randomly-distributed structures, particularly
vertical carbon nanotube arrays. Problems with carbon nanotube array electrodes are the poor nanotube
bonding to
substrates and low tube-to-tube
charge transfer efficiency. The nanotube orientation is easily disrupted, resulting in poor
mechanical robustness, high
internal resistance, and poor
cyclic stability, all a consequence of the weak
van der Waals forces that bind the nanotubes together.[2]
To produce an improved carbon nanoscale electrode architecture, the research team was inspired by tree leaves, which were designed by
nature to have a high surface area for
carbon dioxide absorption for
photosynthesis.[3] Their material design had bio-inspired
micro-conduits in which carbon nanotube arrays serve as branches, and graphene plates as leaves.[2] The graphene plates had large surface area, sharp edges,
mechanical strength, and high electrical conductivity, all features that are desirable in an electrode material.[2] Says
Tim Fisher,
principal investigator of this study and a
professor of
mechanical and aerospace engineering at UCLA,
"We often find inspiration in nature, and plants have discovered the best way to absorb chemicals such as carbon dioxide from their environment... In this case, we used that idea but at a much, much smaller scale - about one-millionth the size, in fact."[3]
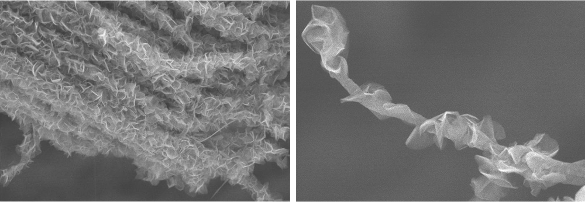
The nanoscale carbon electrode has a branch-and-leaves design incorporating arrays of hollow, cylindrical carbon nanotubes as 'branches,' and sharp-edged, petal-like graphene structures as 'leaves.' (Left image, and right image, from the UCLA Henry Samueli School of Engineering of Applied Science)
The electrode, created by a two-step
microwave plasma chemical vapor deposition process, has highly oriented carbon nanotube array walls decorated by graphene.[2] The nanotubes allow a fast
diffusion of ions during
charge/discharge cycles, while the graphene petals significantly enhance mechanical robustness of the assemblage.[2-3] The hollow, cylindrical carbon nanotubes, are about 20 to 30
nanometers in
diameter, and the graphene petal-like structures are about 100 nanometers wide.[3] The tunnel-shaped arrays allow ions to transport stored energy flow with much less resistance between the
electrolyte and the surface than if the electrode surfaces were flat.[3]
The electrode design provides the same amount of energy storage as similar electrodes, but it's smaller and lighter.
Experiments showed that it produced 30 percent better capacitance for its mass than similar carbon materials, and 30 times better capacitance per area. For these reasons it produced 10 times more
power and retained 95 percent of its initial capacitance after more than 10,000 charging cycles (see graphs).[3] The areal capacitance was 2.35 Farad/cm
2, which works out to be about 500 Farads per
gram, and there was a capacitance retention of about 95% over 10,000 cycles.[2-3]
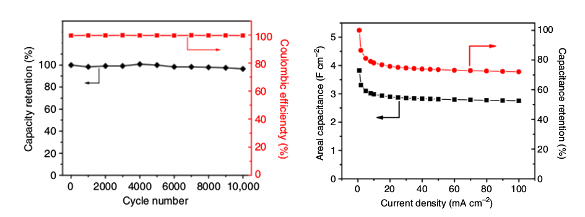
Left, charge/discharge cyclic stability of the electrode at a current density of 60 mA/cm2 and coulombic efficiency. Right, areal capacitance and capacitance retention as a function of current density, as calculated from charge/discharge curves. (Left, fig. 2d, and right, fig. 3d, from ref. 2, licensed under the Creative Commons Attribution 4.0 International License.[2])
It appears that the sharp edges of the graphene increase charge storage and aid the rapid movement of electrolyte
ions in the electrodes, thereby leading to improved supercapacitor performance.[2] This research was funded by the
US Air Force Office of Scientific Research. The
computer simulations were carried out on the
High Performance Computing Collaboratory (HPC2) facility at Mississippi State University.[2]
References:
- An English translation of Heron's Metrica is purportedly available as "Codex Constantinopolitanus Palatii Veteris," E.M.Bruins, Editor, vol 3, (Brill, 1964).
- Guoping Xiong, Pingge He, Zhipeng Lyu, Tengfei Chen, Boyun Huang, Lei Chen & Timothy S. Fisher, "Bioinspired leaves-on-branchlet hybrid carbon nanostructure for supercapacitors," Nature Communications, vol. 9 (February 22, 2018), article no. 790, doi:10.1038/s41467-018-03112-3. This is an open access paper with a PDF file available here
- Inspired by nature: Design for new electrode could boost supercapacitors' performance, UCLA Press Release, February 23, 2018.
Linked Keywords: Calculation; triangle; trigonometry; area; polygon; plane; planar; circle; rectangle; millennia; Archimedes (c.287 BC - c.212 BC); surface area of a sphere; manuscript; On the Sphere and Cylinder; calculus; mathematics; formula; triangle base; triangle altitude; Heron's formula; mathematician; engineer; invention; inventor; steam engine; Aeolipile; ninth century; parameter; semiperimeter; perimeter; decade; material; catalysis; heterogeneous catalysis; gas; liquid; molecule; solid; research; catalyst; adsorption; adsorb; Nobel Laureate; Irving Langmuir (1881-1957); Langmuir adsorption model; Langmuir isotherm; Nobel Prize in Chemistry; book; Nobel Prize; General Electric Research Laboratory; Schenectady, New York; science fiction; author; Kurt Vonnegut (1922-2007); fiction; fictional; ice-nine; phase; water; ice-IX; crystallization; crystallize; Wikimedia Commons; chemisorption; chemical adsorption; physisorption; physical adsorption; temperature; isothermal process; isotherm; partial pressure; Cartesian coordinate system; graph; Brunauer–Emmett–Teller (BET) isotherm; inert gas; chemist; Stephen Brunauer (1903-1986); Paul Hugh Emmett (1900-1985); physicist; Edward Teller (1908-2003); United States Navy; McCarthyism; politics of the United States; J. Robert Oppenheimer (1904-1967); Oppenheimer controversy; security clearance; Gnumeric; electrode; battery; electric double-layer capacitor; supercapacitor; energy storage; parallel plate capacitor; capacitance; relative permittivity; dielectric constant; vacuum permittivity; permittivity of free space; farad; meter; voltage; joule; activated carbon; activated charcoal; electrical conductor; conductive; electrolyte; cubic inch; electrochemistry; electrochemical; chemical decomposition; volt; ionic liquid; electrical polarity; negative; electric charge; positive charge; material; cost; inexpensive; carbon; scientist; engineer; Purdue University (West Lafayette, Indiana); University of Nevada (Reno, Nevada); Central South University (Changsha, China); Mississippi State University (Starkville, Mississippi); Yangtze University (Jingzhou, China); University of California (Los Angeles, California); biomimetics; bio-inspired; leaf; leaves; branch; nanostructure; open access journal; open access paper; Nature Communications; carbon nanotube; nanofiber; graphene; electrical conductor; electrically conductive; randomness; randomly-distributed; vertically aligned carbon nanotube arrays; chemical bond; bonding; wafer; substrate; charge transfer coefficient; charge transfer efficiency; resilience; mechanical robustness; internal resistance; charge cycle; cyclic stability; van der Waals force; nature; carbon dioxide; photosynthesis; micro-conduit; mechanical strength; Tim Fisher; principal investigator; professor; mechanical and aerospace engineering at UCLA; plant; chemical compound; environment; nanoscopic scale; nanoscale; cylinder; cylindrical; UCLA Henry Samueli School of Engineering of Applied Science; microwave; plasma; chemical vapor deposition process; diffusion; nanometer; diameter; electrolyte; experiment; electric power; gram; rechargeable battery; charge/discharge cycle; current density; coulomb; coulombic; efficiency; capacitance; Creative Commons Attribution 4.0 International License; ions; US Air Force Office of Scientific Research; computer simulation; High Performance Computing Collaboratory (HPC2) facility.