Rudolf Clausius
November 19, 2014
Readers of this
blog may have noticed my special fondness for
thermodynamics. This is a consequence of my learning from the excellent introductory
textbook, "Heat and Thermodynamics," by
Mark Zemansky (1900-1981), which went through many
editions. I used the fifth edition, 1968, which is now available online for free download, courtesy of the
Internet Archive.[1] Zemansky's textbook was excellent preparation for subsequent
courses in
statistical mechanics and
solid state thermodynamics in
graduate school.
Zemansky, who was a
professor of
physics at the
City College of New York, is noted also as the
coauthor of the the introductory physics textbook, "University Physics." This was written with
Francis Sears, and it was better known as "Sears and Zemansky," but I used the massive "
Resnick and
Halliday," instead.[2] I met Zemansky when he toured our
Upstate New York physics department in the late
1960s.
Most
scientists are familiar with the
"laws" of thermodynamics. It's especially interesting that after years of having three laws, it was decided that something fundamental was missing, so a
"zeroth" law was added. This seems
analogous to the problem of the
parallel postulate in
Euclidean geometry; but, fortunately, adding this law didn't give us more than one
classical thermodynamics. Here's a short summary of the four laws.
Zeroth Law of Thermodynamics
The zeroth law of thermodynamics states that two thermodynamic systems in thermal equilibrium with a third will be in thermal equilibrium with each other; that is, all three will be in thermal equilibrium. British physicist, Ralph H. Fowler, saw the need for this law in 1935, long after the other laws were codified.
First Law of Thermodynamics
The first law of thermodynamics is essentially an energy conservation law. It states that the change in the internal energy of an isolated system is equal to the amount of heat supplied to the system, minus the amount of work done by the system on its surroundings. This law says that perpetual motion machines of the first kind are impossible, since these produce work without any input energy.
Second Law of Thermodynamics
The second law of thermodynamics states that the entropy of an isolated system will never decrease. Systems are always moving towards thermodynamic equilibrium, which has maximum entropy. This is the law that disallows perpetual motion machines of the second kind. Such machines would do work merely by cooling a thermal reservoir to extract its heat. A valid heat engine must operate against a temperature difference between hot and cold reservoirs.
Third Law of Thermodynamics
The third law simply states that the entropy of a perfect crystal is exactly zero at absolute zero. However, this statement is usually turned around to mean that if you don't have zero entropy, you aren't at absolute zero. Zero entropy in an actual material appears to be impossible to obtain, so this law essentially states that you can never reach absolute zero.
Of all these laws, the second law is the most interesting, since it deals with the concept of entropy.
Natural processes are
irreversible, so the entropy of the world is always increasing. We can make a small patch of order, here or there, as I did when I
grew crystals in the
laboratory, but only at the expense of order somewhere else. The
German physicist,
Rudolf Clausius (1822-1888) is responsible for much of our understanding of the second law.

Rudolf Clausius (1822-1888)
In an 1865 paper, Clausius wrote the following simplified statements of the first and second laws: the energy of the universe is constant; the entropy of the universe tends to a maximum.[4]
(Cropped photograph by Theo Schafgans (1859–1907) from the Zeitschrift für Physikalische Chemie, vol. 21, 1896, scanned by Armin Kübelbeck, via Wikimedia Commons.)
Clausius' first major dip into the heat reservoir was his 1850 paper, Über die bewegende Kraft der Wärme," (On the Moving Force of Heat), in which he restated the first and second laws in a more logical fashion. Clausius first touched on the second law concept in 1854, when he wrote, "Heat can never pass from a colder to a warmer body without some other change, connected therewith, occurring at the same time."[3] What is meant by this statement is that a
mechanical agent is required to make, for example, a
refrigerator. Something doesn't get cooler if we just let it sit there.
In an 1865 paper, Clausius gave entropy its name, from the
Greek,
εν (in accordance with) plus
τροπη (a change).[4] As Clausius writes,
"I have intentionally formed the word entropy so as to be as similar as possible to the word energy; for the two magnitudes to be denoted by these words are so nearly allied in their physical meanings, that a certain similarity in designation appears to be desirable."[4-5]
Thermodynamics is generally concerned with cycles, such as the simplified version of a
heat engine known as the
Carnot cycle.
Carnot proposed this cycle in 1824, but it was Clausius who analyzed it in terms of entropy in 1862 in what's now known as the
Clausius theorem; viz.,
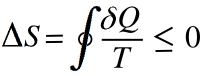
in which
δQ is the amount of heat absorbed by the system and the
integral is over the heat engine cycle. The
inequality states that in a
reversible process, the entropy change
ΔS is zero; but, if the process is
irreversible, as most everything is, there will be a change in entropy. The signs are defined so that a loss of heat is negative, making the entopy change negative.
There's the curious fact that entropy is always
symbolized by the letter "S." In a 2001 paper,
Irmgard K. Howard of the
Department of Chemistry,
Houghton College (Houghton, NY), explained that Clausius was using "S" to symbolize entropy long before he had a name for it, and the symbol stuck. That was also the case for using "U" to symbolize energy, another confusion for
students of thermodynamics.[5]
As a
materials scientist, I've often made use of another of Clausius' equations, the
Clausius-Clapeyron relation, which is a way to estimate the
vapor pressure of a
material when its
latent heat of vaporization L is known. The equation, as it's usually written, is
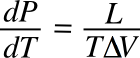
which brings together
pressure P,
temperature T, and
volume V, all in one equation. I was more likely to use it in a converse sense, to estimate the latent heat from the other quantities.
References:
- Mark W. Zemansky, "Heat and Thermodynamics," 5th.ed., McGraw-Hill Book Company Inc., 1968 (Free download via archive.org).
- Robert Resnick and David Halliday, "Physics for Students of Science and Engineering," 7th ed., John Wiley & Sons, 1965. Sorry, no free downloads available for this title.
- R. Clausius, "Über eine veränderte Form des zweiten Hauptsatzes der mechanischen Wärmetheorie," Annalen der Physik, vol. xciii (1854), p. 481ff. Translated into English as R. Clausius, "On a Modified Form of the Second Fundamental Theorem in the Mechanical Theory of Heat," The London, Edinburgh and Dublin Philosophical Magazine and Journal of Science, 4th ed., vol. 2 (July 1856), p. 86.
- R. Clausius, Ninth Memoir (1865), in "The Mechanical Theory of Heat, with its Applications to the Steam-Engine and to the Physical Properties of Bodies," T. Hirst, Ed., John Van Voorst, London, 1867 (via Google Books).
- Irmgard K. Howard, "S Is for Entropy. U Is for Energy. What Was Clausius Thinking?" Journal of Chemical Education, vol. 78, no. 4 (April, 2001), pp. 505-508. A PDF file is available, here.
Permanent Link to this article
Linked Keywords: Blog; thermodynamics; textbook; Mark Zemansky; edition; Internet Archive; course; statistical mechanics; solid state; graduate school; professor; physics; City College of New York; coauthor; Francis Sears; Robert Resnick; David Halliday; Upstate New York; 1960s; scientist; laws of thermodynamics; zeroth law of thermodynamics; analogy; analogous; parallel postulate; Euclidean geometry; classical thermodynamics; thermodynamic system; thermal equilibrium; British; Ralph H. Fowler; first law of thermodynamics; energy conservation; internal energy; isolated system; heat; work; perpetual motion machines of the first kind; second law of thermodynamics; entropy; perpetual motion machines of the second kind; thermal reservoir; heat engine; third law of thermodynamics; crystal; absolute zero; nature; natural; irreversible process; crystal growth; laboratory; German; Rudolf Clausius (1822-1888); energy; universe; entropy; Wikimedia Commons; mechanical agent; refrigerator; Greek language; heat engine; Carnot cycle; Sadi Carnot; Clausius theorem; integral; inequality; reversible; irreversible; symbol; symbolize; Irmgard K. Howard; Department of Chemistry; Houghton College (Houghton, NY); student; materials scientist; Clausius-Clapeyron relation; vapor pressure; material; latent heat of vaporization; pressure; temperature; volume.