Scientific Thought
May 6, 2011
Dilbert is a composite of many technologists. He often functions as a
computer scientist who's knowledgeable in both
hardware and
software. Sometimes he acts more like a "
rocket scientist," inventing strange and wonderful devices. What's apparent is that he thinks differently than people in general, which might explain his problem in attracting women. This is true, also, of the
scientists and
engineers on the television series, "
The Big Bang Theory." Their
analytical mode of thought is quite unlike the
wishy-washy musings of those around them, so they're often unintentionally insulting.
I took a course in
physics when I was a
high school senior. This wasn't an ordinary course, since we were the
Sputnik-response generation. It was
PSSC Physics, which was designed to teach not just the
F = ma style facts, but how physicists think.[1] There was a textbook with questions at the end of each chapter, and I was called upon to answer the first question at the end of the first chapter. Now, how hard could question one of chapter one be?
The question was, why does a
light ray bend when it enters
water. My response was, "I don't know." Our teacher, who likely wondered how this sort of student got into his PSSC physics class, replied that it was because of
Snell's Law. I don't remember whether I stifled a laugh, but Snell's Law certainly isn't the reason why. It's merely a description of what happens. It was quite a bit later that I learned "why" light bends when it enters a region of higher
refractive index.
I was reminded of the difference between a description and an explanation while reading a book review in
American Scientist.[2] The reviewer described a scene from
Molière's comedy,
The Imaginary Invalid (Le Malade imaginaire) in which the physicians explained that
opium causes sleep because of its "dormitive power." The parallel to my Snell's Law example is easy to see. We should be careful to know the difference between a description and an explanation.
Scientists, in their honesty, can be abrasive. I once shared a coffee break at a topical conference with a somewhat famous physicist, the inventor of an important scientific instrument. This was just after a presentation of some interesting results by a
physical chemist. The chemist had made the mistake of proffering an explanation of his experimental results.
Well, according to my coffee mate, this guy didn't know the difference between an
electron and a sunny day, all because he framed his explanation in the language of chemistry, and not of physics. I think the chemist's explanation satisfied 95% (
two sigma) of the audience, but it makes you wonder what an explanation really entails.
The idea of explanation is easier in
mathematics; in fact, it's codified in the definition of a theorem. You start with axioms, the truth of which are (
generally) inarguable, and you build to a more complex and satisfying conclusion. That's one reason why physics is so mathematical and why physicists believe that their conception of the world is better than yours.
Theory, the stuff of explanation, is essential when
extrapolation is required, but most of our world experiences are not extrapolations, they're
tautologies. The answer to nearly all everyday questions can be found in
Wikipedia.
Vannevar Bush had this same idea when he wrote the article, "
As We May Think," in the
Atlantic Monthly.[3] I wrote about Bush in a
previous article (Basic Research, October 22, 2010). His mechanized
hypertext machine, called
Memex, is much like Wikipedia, and Bush thought that having such a device available for research would make science more efficient. His article also mentioned devices similar to
Mathematica for solving and resolving
equations, another example of tautology. Let's face it, most of the world doesn't need an explanation, it just needs a list of examples and a crank to turn.
Thomas Kuhn, in his influential 1962 book,
The Structure of Scientific Revolutions, wrote about "normal" science. Normal science is what's practiced by nearly every scientist. Normal science is essentially the Snell's Law type of science in which we hone better predictions of extant theories. This is akin to adding more
epicycles to our description of
planetary orbits as more data become available.
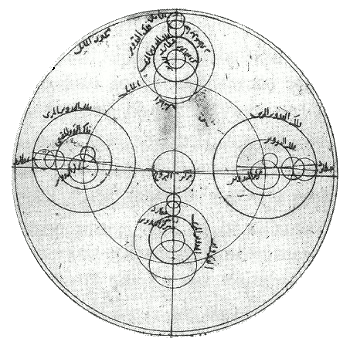
Ibn al-Shatir's 14th century model for the orbit of Mercury using Ptolemaic epicycles.
(Via Wikimedia Commons)
Eventually, some scientist gets tired of adding more epicycles, and he finds a better explanation; namely, that planets revolve around the
Sun, not the
Earth. There's push-back from the normal scientists who've invested their careers in epicycles, but there's an eventual
paradigm shift.
Newton's Law of Universal Gravitation may have described planetary orbits quite well, but we still needed to wait for
General Relativity for the explanation as to
why the planets revolve around the Sun. All my science has been "normal," but I still know a good explanation when I see one.
References:
- I had a physics teacher who told his class that all of physics was F = ma. You just needed to figure out what was meant by F, m and a in each particular case, as when talking about the effective mass of an electron.
- Cosma Shalizi, "The Domestication of the Savage Mind," Review of the book, WHAT IS INTELLIGENCE? Beyond the Flynn Effect (James R. Flynn, Cambridge University Press, 2009), American Scientist, vol. 97, no. 3 (May-June 2009), pp. 244ff.
- Vannevar Bush, "As We May Think," The Atlantic Monthly, July, 1945.
Permanent Link to this article
Linked Keywords: Dilbert; computer scientist; hardware; software; rocket scientist; scientist; engineer; The Big Bang Theory; analytical; wishy-washy; physics; high school senior; Sputnik; PSSC Physics; light ray; water; Snell's Law; refractive index; American Scientist; Molière; The Imaginary Invalid; opium; physical chemist; electron; normal distribution; two sigma; mathematics; parallel postulate; theory; extrapolation; tautology; Wikipedia; Vannevar Bush; As We May Think; Atlantic Monthly; basic research; hypertext; Memex; Mathematica; equation; Thomas Kuhn; The Structure of Scientific Revolutions; epicycle; planetary orbits; Ibn al-Shatir; Wikimedia Commons; Sun; Earth; paradigm shift; Newton's Law of Universal Gravitation; General Relativity; effective mass of an electron.