Eratosthenes
March 17, 2011
When I was a child, my parents must have noticed my
mathematical bent, since I received as a
Christmas present one year "The Giant Golden Book of Mathematics."[1] This oversized book (10-1/2 inches wide by 12 inches tall) still sits on my bookshelf as an inspiration. It's also a reminder that dead-tree books are better than
e-books in many cases. This book has one chapter, "Numbers We Cannot Split," about
prime numbers and the
Sieve of Eratosthenes.
Eratosthenes (c. 276 BC - c. 195 BC) was a
Greek mathematician and a friend of
Archimedes. He's best know for the prime number sieve, which is understandable to most schoolboys. The sieve has been improved somewhat over the years, the improvements being in the means of quickly eliminating the non-primes (
composite numbers) from the
integers. Eratosthenes has us just cross out every number divisible by two, then three, five, etc., and this method will, of course, work. A program to do this is easy to write, and I have some source code
here.

Eratosthenes at work. Engraving after a work by the French painter Gustave Courtois (1853-1923), via arXiv.[2]
An Indian schoolboy, S. P. Sundaram, perhaps inspired by the role model of
Ramanujan, found
a better technique in 1934.[3] To find all primes up to about 2n,
Sundaram's sieve has us cast out all numbers (i + j + 2ij), where i and j are positive integers, (i + j + 2ij) is less than or equal to n, and i < j. The remaining numbers are then multiplied by two and added to one. This gives you all prime numbers (except for 2) below 2n + 2. A
Canadian high school student, Bai Li, writes a
very nice blog, and he has posted some code that uses Sundaram's technique.[4]
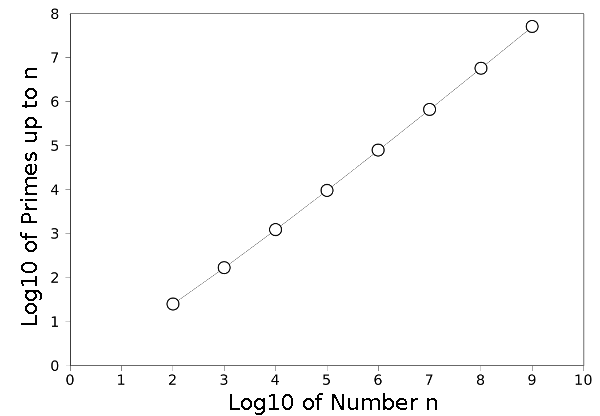
Number of primes less than n. A log10-log10 scale is used with data from my primes program. Many more values can be found here. Graph rendered using Gnumeric.
The idea that, on a lark, I can write a program to find the first 100 million prime numbers, shows how far we've come from the "Golden Book" days. The figure above shows one of the regularities of prime numbers that makes them interesting. However, prime number theory has advanced well beyond mere
lists of primes, and it's too big a topic for a blog article; so I'll mention another discovery of Eratosthenes. He measured the
size of the Earth quite accurately more than two millennia ago.
Pliny the Elder, whom I've mentioned quite a few times in previous articles, reported on Eratosthenes'' measurement.[5]
And thus much of the length and breadth of the earth, which I thought worth the writing. Now the universall compasse and circuit thereof, Eratosthenes (a great Clerke verily for all kind of literature, and in this knowledge above all others doubtlesse most cunning, and whome I see of all men approved and allowed) hath set downe to be 252000 stadia. Which measure, by the Romanes account and reckoning, amounteth to 300 hundred and 15 hundred miles. A wonderous bold attempt of his! but yet so exquisitely calculated and contrived by him, that a shame it were not to beleeve him.
Pliny had a way with words, but not much for mathematical exposition. Khristo N. Boyadzhiev, in an article posted to
arXiv last year,[2] interprets the text as saying that
Earth's circumference is 39,375 km, which is extremely close to the actual equatorial value of 40,075 km. Pliny didn't quite believe that such a value could be calculated. Boyadzhiev writes that Pliny thought you would need to travel to Earth's center to judge its radius, and this practical outlook was the reason why there was no tradition of science in
Ancient Rome.
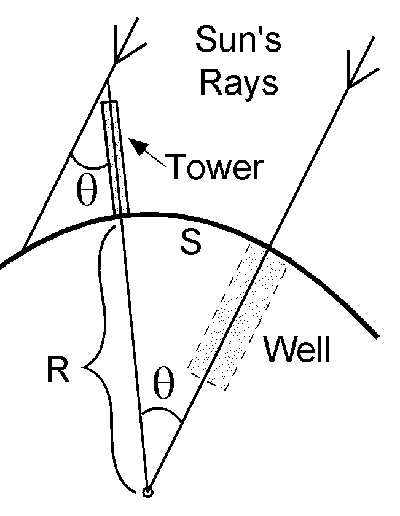
Eratosthenes' measurement of Earth's radius.
The angle θ is 7.2 degrees, and the circle arc S is 787.5 km)
Eratosthenes'' method was really very simple, as the figure shows. He knew that on June 22 of each year, the
Sun was directly above a particularly deep
well in Siena,
Egypt. This was known, since the Sun could be seen from the bottom of the well. Eratosthenes knew that the Sun, the well and the Earth's center would need to be on a straight line at that particular time for this to happen. He also knew that a 120 meter tower in
Alexandria cast a shadow that showed that the Sun's angle at that point on Earth was 7
o 12' (7.2 degrees), which is 1/50 of 360-degrees (He probably rounded his data for that to happen). Note that his calculation assumes that the Sun is very far away; that is, the Sun's rays are roughly
parallel.
From simple geometry, we see that the angle between the tower and the well, with the Earth's center as the apex, is also 7.2 degrees, and we can use the circle arc formula for finding Earth's radius, knowing that the distance between the tower and well is 5000 Egyptian stadia, or 787.5 km.
R = S/θ,
where θ is in radians. For 7.2 degrees, this is 0.1256637 radians, giving a radius (R) of 6,266.7259 km and a circumference of 39,374 km.
There are some "
urban legends" about
Christopher Columbus, one of which is that he made his
historic voyage to prove the world was round. It's fairly certain that Columbus knew about Earth's spherical shape, but he didn't have a good idea of its size. His intent was to sail to
India by going west, instead of east; instead, he bumped into the
Americas and called the inhabitants "
Indians." Perhaps if he had read Eratosthenes, he would have known better. Then again, if he had known better, he might not have made his trip.
References:
- Irving Adler, "The Giant Golden Book of Mathematics," Illustrated by Lowell Hess, Golden Press (New York, 1960), 92 pages.
- Khristo N. Boyadzhiev, "Eratosthenes and Pliny, Greek geometry and Roman follies," arXiv Preprint, June 15, 2010.
- Sieve of Sundaram page on Wikipedia.
- Sieve of Sundaram article on Bai Li's blog.
- Philemon Holland, translator (1601): G. Plinius Secundus The Historie of the World, Book II, Paragraph 247.
De longitudine ac latitudine haec sunt, quae digna memoratu putem. universum autem circuitum Eratosthenes, in omnium quidem litterarum subtilitate, set in hac utique praeter ceteros solers, quem cunctis probari video, CCLII milium stadiorum prodidit, quae mensura Romana conputatione efficit trecentiens quindeciens centena milia passuum: inprobum ausum, verum ita subtili argumentatione conprehensum, ut pudeat non credere.
- Chris Caldwell, "Online prime test," Prime Curios! web site at The University of Tennessee at Martin.
Permanent Link to this article
Linked Keywords: Mathematics; Christmas; e-book; prime number; Sieve of Eratosthenes; Eratosthenes; Greek; mathematician; Archimedes; composite number; integer; Gustave Courtois; Srinivasa Ramanujan; Sieve of Sundaram; Canadian; high school; luckytoilet.wordpress.com; primes program; Gnumeric; lists of primes; geodesy; size of the Earth; Pliny the Elder; arXiv; Earth; circumference; Ancient Rome; Sun; well; Egypt; Alexandria; parallel; urban legend; Christopher Columbus; historic voyage; India; Americas; Native Americans; Indians; Khristo N. Boyadzhiev.