Auxetics
May 13, 2011
Some
scientific discoveries are completely counter-intuitive. Having been presented with example after example of how something works, you're suddenly thrown a
Black Swan. This is all the more embarrassing when you find that the theory you've been using all these years actually allows Black Swans. You just weren't looking that hard for them. The
positron is one example.
Auxetic materials are another.
First, some background. Materials have a certain mechanical constant called
Poisson's ratio, which is usually symbolized by the symbol,
ν. This constant is the measure of how much the material will shrink in a plane when you pull on it. Technically, it's the ratio of the contraction in the x- or y-direction to the extension in the z-direction. There's a negative sign thrown in so that the ratio is positive for normal materials, which is everything from
marshmallows to
molybdenum.
Poisson' ratio is good for more than designing
automobile engines. When I worked with thin,
crystalline films, an important thing to know was the stress state of the film on its substrate. Using
xray diffraction, it was easy to get the
lattice constant of the film in the direction perpendicular to the plane, but what we really wanted was its value in the plane. Knowing the Poisson' ratio for these materials allowed that calculation, and it was then a simple comparison between that value and the value for the substrate to determine the film
strain.
Our usual experience is that materials get thinner as we pull on them, and Poisson's ratio is usually in the range of about 0.3 for most metals.
Cork is interesting, since its Poisson's ratio is about zero, which makes it ideal for its bottle stopper application, since it can be inserted and removed easily. Cork doesn't expand when pushed into the bottle neck, so it doesn't jam.
Rubber is unusual in the other sense, since it has a very large Poisson's ratio about 0.5. So much for "normal" materials. There are other materials with the counter-intuitive property of fattening when they're pulled; that is, they have a negative Poisson's ratio.
Every good
phenomenon must have a
Greek name, although the supply of classics scholars is dwindling. Fortunately,
Ken E. Evans of the University of Exeter stepped up to the plate with a name for these materials,
auxetics, derived from αυξητικος (auxetikos), meaning "something that tends to increase." If the word seems familiar, it's because "auxiliary" is derived from the same stems.
Auxetic materials hit the scientific scene with a paper published in
Science in 1987.[2] This publication especially excited
one of my coworkers, who launched a research project in the area. The utility of such materials is limited because they have low
density; indeed, this first paper described a
foam. The low density is required to accommodate all the internal mechanical contortions required for this effect. You can see this in the model system that appears in the figure.
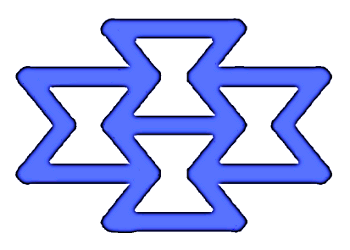
Two-dimensional model of an auxetic material. Pulling it from the top and bottom will cause the sides to expand, which is opposite to a "normal" material's response)
The model system is a deformed
hexagonal lattice of elastic links embedded in a somewhat empty space. You can make one of these by squirting
silicone into this pattern onto waxed paper to see that pulling it from the top and bottom will cause the sides to expand. It's easier to just see an animation.[3] Of course, there's the problem of what constitutes a material, and what constitutes a
simple machine. You can make this in
nano form and continue to shrink it, but at what point does it stop being a machine and becomes a material?
The foam studied in the first auxetic material article was a
polyurethane foam with a three dimensional honeycomb structure. The honeycombs were then reduced to a buckled structure, like the one shown in the figure, using high pressure.
Rod Lakes, then at the
University of Iowa and author of this first paper, later found a different auxetic principle. This other auxetic principle, involving nodules connected by fiber strands, was found in the
Gore-Tex version of
polytetrafluorethylene.
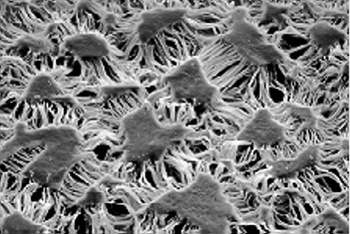
Electron micrograph of a Gore-Tex membrane. Nodule size is about 10μm.
(via Wikimedia Commons)
Auxetic materials are useful for
impact resistance. When a normal material is indented, it spreads away from the indenter. Auxetic materials have the opposite response - they move towards the indent and act to block the indenter.
References:
- Rod Lakes, "Negative Poisson's ratio materials," University of Wisconsin web page.
- Roderic Lakes, "Foam structures with a negative Poisson's ratio", Science, vol. 235, no. 4792
- Auxetic Material Animation, Auxetic Materials Network.
- Maria Burke, "A stretch of the imagination," New Scientist, vol. 154, no. 2085 (June 7, 1997), pp. 36 ff. Another version of the article appears here.
Permanent Link to this article
Linked Keywords: Scientific; Black Swan; positron; auxetic materials; Poisson's ratio; marshmallows; molybdenum; internal combustion engine; crystal; crystalline; xray diffraction; lattice constant; deformation; strain; Cork; rubber; phenomenon; Greek language; Ken E. Evans; auxetics; Science; Ray H. Baughman; density; foam; hexagonal lattice; silicone; simple machine; nanotechnology; polyurethane; Rod Lakes; University of Iowa; Gore-Tex; polytetrafluorethylene; Wikimedia Commons; impact resistance.